Choques en 1 dimensión¶

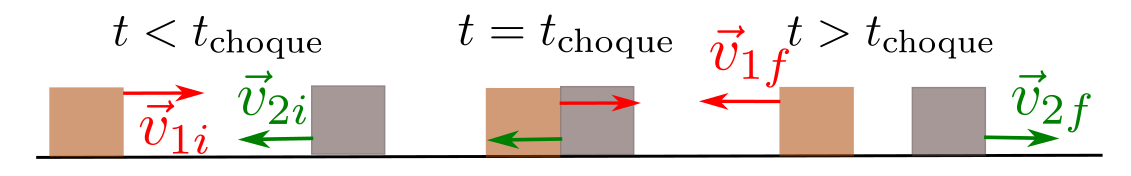
Fig. 130 In a 1 dimensional collision two masses, with initial velocities \(v_{1i}\), \(v_{2i}\) interact and, as a result, have final velocities \(v_{1f}\) and \(v_{2f}\).¶

Inelastic collision¶

(270)¶\[m_1 v_{1i} + m_2 v_{2i}=m_1 v_{1f} + m_2 v_{2f}\]

(271)¶\[m_1 v_{1i} + m_2 v_{2i}=m_1 v_{1f} + m_2 v_{2f}=(m_1+m_2)\vec{v}_f \rightarrow \vec{v}_f = \frac{m_1 v_{1i} + m_2 v_{2i}}{m_1+m_2}\]

Elastic collision¶

(272)¶\[m_1 v_{1i} + m_2 v_{2i}=m_1 v_{1f} + m_2 v_{2f}\]

(273)¶\[\frac{1}{2}m_1 v_{1i}^2 + \frac{1}{2}m_2 v_{2i}^2=\frac{1}{2}m_1 v_{1f}^2 + \frac{1}{2}m_2 v_{2f}^2 \rightarrow m_1 (v_{1i}-v_{1f})(v_{1i}+v_{1f}) = m_2 (v_{2f}-v_{2i})(v_{2f}+v_{2i})\]

(274)¶\[v_{1i}+v_{1f}=v_{2f}+v_{2i} \rightarrow v_{1i}-v_{2i}=-(v_{1f}-v_{2f})\]

(275)¶\[\begin{split}v_{1i}-v_{2i}=-(v_{1f}-v_{2f}) \longrightarrow \left\{\begin{aligned}v_{1f}=v_{2f}+v_{2i}-v_{1i}\\v_{2f}=v_{1f}+v_{1i}-v_{2i}\end{aligned}\right.\end{split}\]

(276)¶\[v_{1f}=\frac{m_1-m_2}{m_1+m_2}v_{1i}+\frac{2m_2}{m_1+m_2}v_{2i}\]

(277)¶\[v_{2f}=\frac{2m_1}{m_1+m_2}v_{1i}+\frac{m_2-m_1}{m_1+m_2}v_{2i}\]

Particles with the same mass¶

(278)¶\[v_{1f}=\frac{m_1-m_2}{m_1+m_2}v_{1i}+\frac{2m_2}{m_1+m_2}v_{2i}=v_{2i}\]

(279)¶\[v_{2f}=\frac{2m_1}{m_1+m_2}v_{1i}+\frac{m_2-m_1}{m_1+m_2}v_{2i}=v_{1i}\]

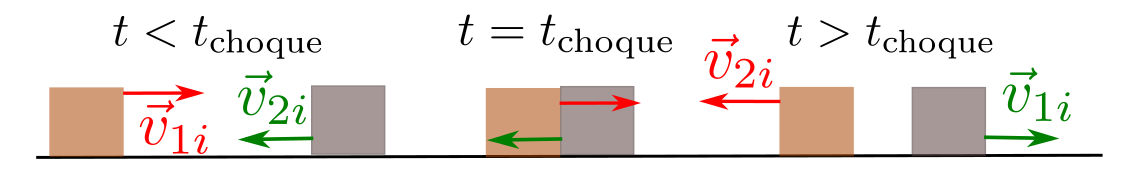
Fig. 131 In a 1 dimensional collision when the mass of both bodies is the same, the initial and final velocities of the particles are exchanged.¶

A particle is much heavier than the other¶

(280)¶\[v_{1f}=\frac{m-M}{m+M}v_{1i}+\frac{2M}{m+M}v_{2i}\approx-\frac{M}{M}v_{1i}+\frac{2M}{M}v_{2i}=-v_{1i}+2v_{2i}\]

(281)¶\[v_{2f}=\frac{2m}{m+M}v_{1i}+\frac{M-m}{m+M}v_{2i}=v_{1i}\approx 0 v_{1i}+\frac{M}{M}v_{2i}=v_{2i}\]

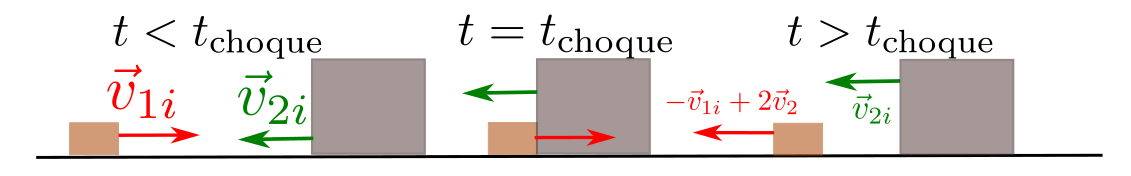
Fig. 132 In a 1 dimensional collision when the mass of one of the bodies is much larger than the other, the lighter one simply bounces off the heavier one.¶

Magnitudes escalares¶

I am writing an equation inline \(x=-i\hbar\psi=\hat{h}\psi\).

If the equation is by itself,

(282)¶\[-i\hbar\psi=\hat{h}\psi\]

I am going to add a figure

Fig. 133 Here is my figure caption!¶

:::{admonition,warning} This is also Markdown This text is standard Markdown :::

:::{admonition,note} This is also Markdown This text is standard Markdown :::

:::{admonition,tip} This is also Markdown This text is standard Markdown :::

Magnitudes vectoriales¶

There are many ways to write content in Jupyter Book. This short section covers a few tips for how to do so.

I am going to cite a reference [HdHPK14]

Now I am going to cite section escalares Sec. Magnitudes escalares

The Schrödinger equation is Eq. (195)

I am citing the figure: Fig. 102

Unidades¶

Problemas y ejemplos resueltos¶

This is the text of a problem

I can start solving like this

(283)¶\[x=-2\pi\]

Some text needs to go between sidebars

(284)¶\[y=-log(e)\]

And at the end

This is the text of another problem

Física General