Vector fields¶
Definition¶
A vector field is a magnitude that attributes a single vector to each of the point of the space where it is defined.
Example
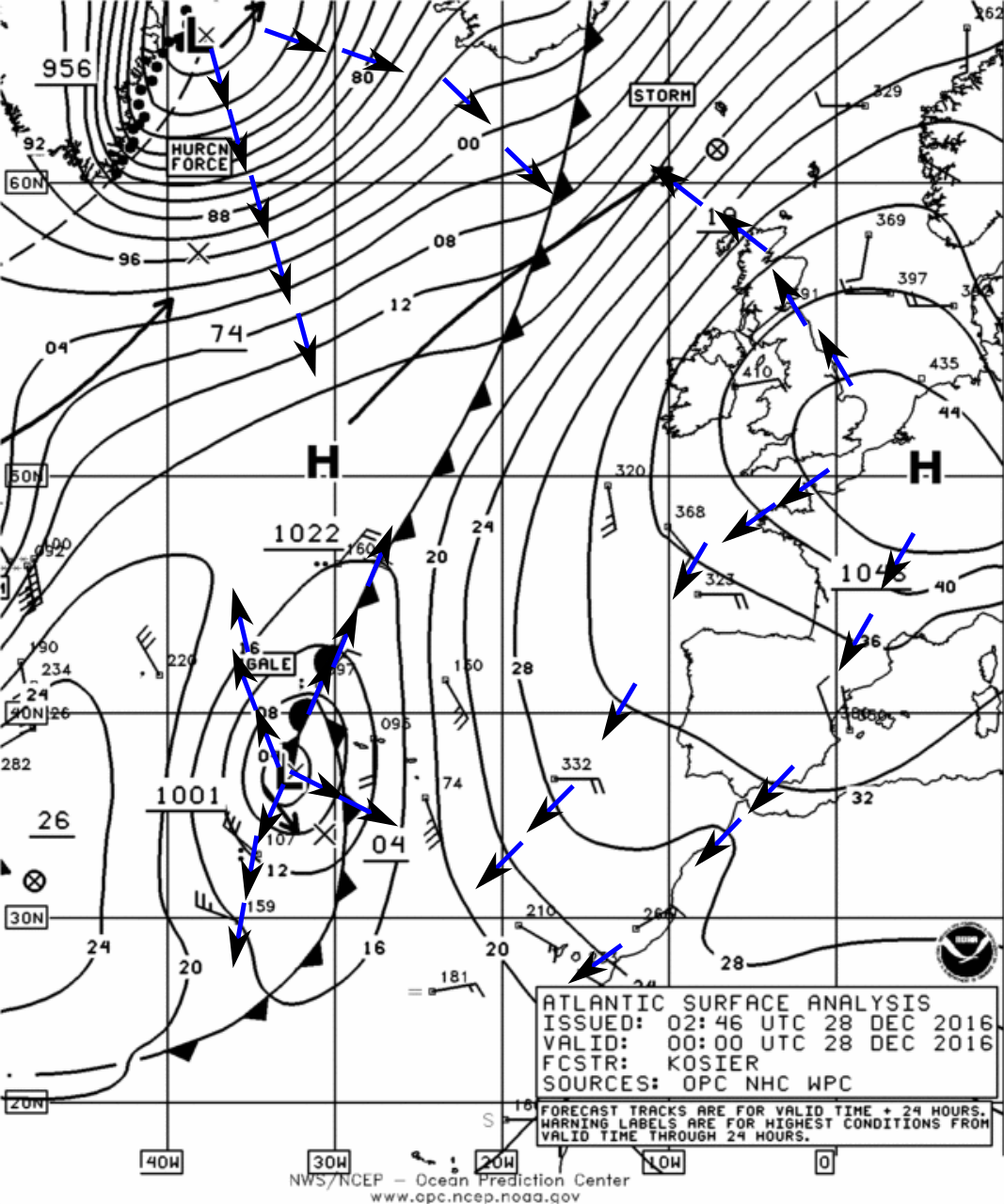
Fig. 23 In the weather report we can observe the wind’s velocity in each point of the map.¶
In the weather report we can usually see a scalar map for atmospheric pressure superimposed with a vector map for the winds. Obviously both quantities are related by the fact that the air will try to go from places where the pressure is higher to the ones where the pressure is lower.
Field lines¶
A very useful representation of vector fields comes in the form of the so-called field lines that are extensively used in several areas of physics but that are particularly important in electromagnetism.
The field lines are, as their name indicates, lines that are travelled in a particular direction (their sense), and that have the following properties:
-Direction: the field lines are tangent to the field in every point.
-Line density: the more lines present around a point the more intense the field is. Conversely, a low density of lines is indicative of a region having a weak field.
-Line crossings: Line crossings can only touch at a source or sink of field lines. In the absence of sources or sinks lines can never touch.
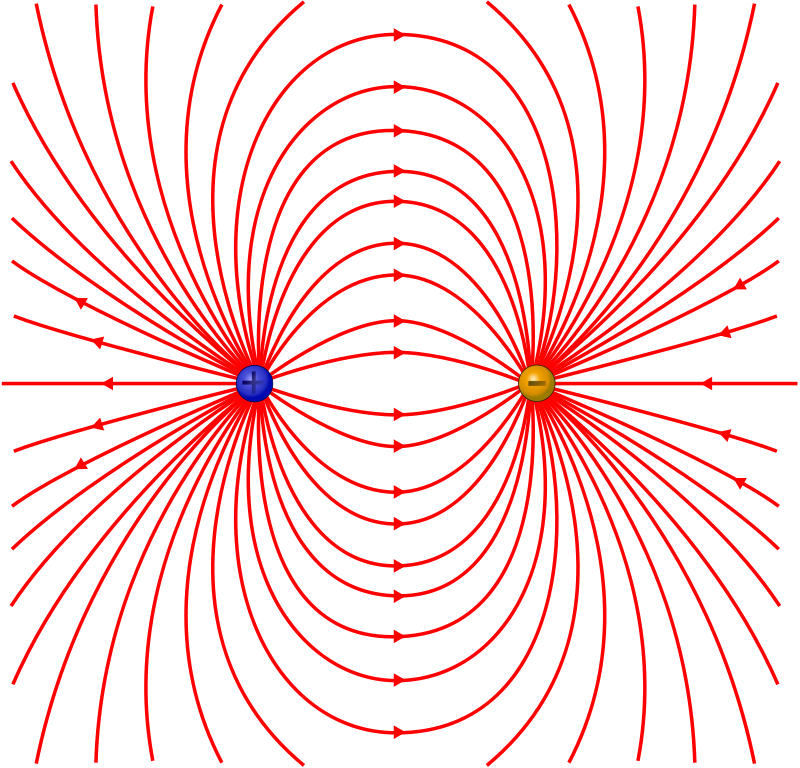
Fig. 24 Illustration of the field lines created by a charge dipole. As can be seen the lines are created at the source (the positive charge) and travel without touching to dissappear at the sink (the negative charge).¶
