Energía potencial¶

Conservative forces¶

Fuerzas conservativas

(213)¶\[\begin{split}W &=\int\vec{F}d\vec{r}\underset{d\vec{r}=dy\vec{j}}{=}\int -mg\vec{j} dy\vec{j}= \\ &= -mg\int_{y_0}^{y_f} dy=-mg\Delta y\end{split}\]

Potential energy¶

(215)¶\[W=\Delta T = - \Delta U\]

Fig. 109 The absolute value of the work carried out by the weight when a mass is lifted or dropped a particular height is the same. If we dropped the mass and the lifted it to the initial position the work carried out by the weight would be null.¶

(215)¶\[W=U(\vec{r}_{\text{initial}}-\vec{r}_{final}}\]

Gravitational energy¶

Fig. 110 The absolute value of the work carried out by the weight when a mass is lifted or dropped a particular height is the same. If we dropped the mass and the lifted it to the initial position the work carried out by the weight would be null.¶

(216)¶\[U=mgy\]

Energy stored in a spring¶

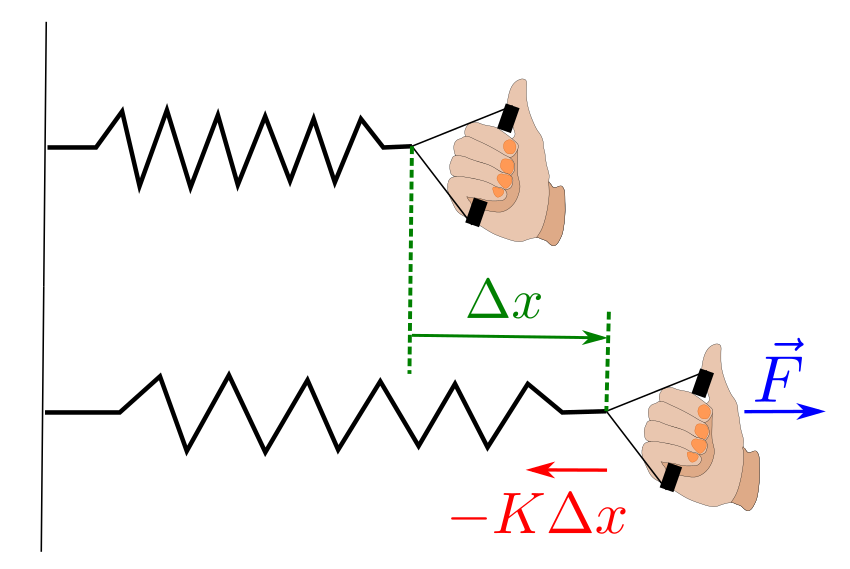
Fig. 111 A spring can store potential energy as it is expanded or compressed.¶

(220)¶\[\vec{F}=-Kx\vec{u}_x\]

(218)¶\[W=\int_{x_0}^{x_f}\vec{F}_\text{aplic}d\vec{r}\underset{d\vec{r}=xdx\vec{i}}{=}\int_{x_0}^{x_f}Kxdx=K \left.\frac{x^2}{2}\right\vert_{x_0}^{x_f}=\frac{1}{2}K(x_f^2-x_0^2)\]

(219)¶\[U=\frac{1}{2}Kx^2\]

Force due to potential energy¶

(220)¶\[\delta W=\vec{F}\cdot d\vec{r} = - dU\]

(221)¶\[\vec{\nabla} U \cdot d\vec{r}=\sum_i \frac{\partial U}{\partial x_i} dx_i = dU = -dW = -\vec{F}d\vec{r} \rightarrow \stress{\vec{F}=-\vec{\nabla}U}\]

(222)¶\[\Delta U = - W = -\int_{\vec{r}_0}^{\vec{r}_f} \vec{F}\cdot d\vec{r}\]

(223)¶\[\vec{F}=-\vec{\nabla}U\]

Magnitudes escalares¶

I am writing an equation inline \(x=-i\hbar\psi=\hat{h}\psi\).

If the equation is by itself,

(224)¶\[-i\hbar\psi=\hat{h}\psi\]

I am going to add a figure

Fig. 112 Here is my figure caption!¶

:::{admonition,warning} This is also Markdown This text is standard Markdown :::

:::{admonition,note} This is also Markdown This text is standard Markdown :::

:::{admonition,tip} This is also Markdown This text is standard Markdown :::

Magnitudes vectoriales¶

There are many ways to write content in Jupyter Book. This short section covers a few tips for how to do so.

I am going to cite a reference [HdHPK14]

Now I am going to cite section escalares Sec. Magnitudes escalares

The Schrödinger equation is Eq. (195)

I am citing the figure: Fig. 102

Unidades¶

Problemas y ejemplos resueltos¶

This is the text of a problem

I can start solving like this

(225)¶\[x=-2\pi\]

Some text needs to go between sidebars

(226)¶\[y=-log(e)\]

And at the end

This is the text of another problem

Física General